Geodetic Coordinates

Geodetic Datum

In geodetic coordinates, the parameters of the ellipse (which represents the Earth or another planetary body in an ellipsoidal model) are specified using the following key parameters:

• $f=\frac{a-b}{a}$

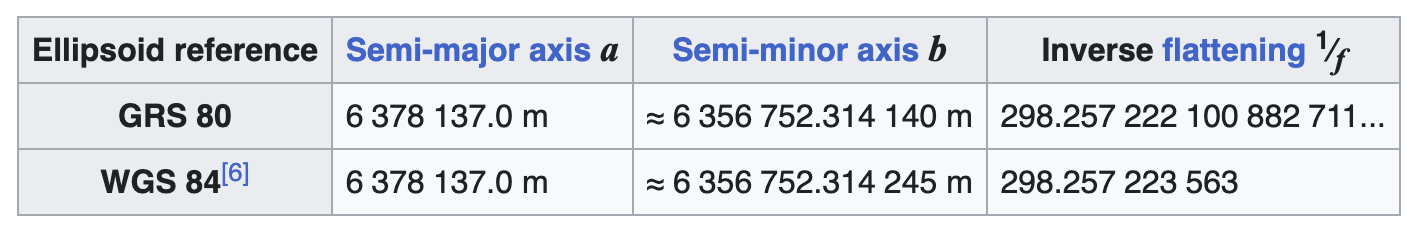
• Where aaa is the semi-major axis and bbb is the semi-minor axis.

Earth is modelled as an oblate spheroid.

• Because it is nearly symmetrical about its rotational axis.

Ellipsoid: The Earth is not a perfect sphere; it’s slightly flattened at the poles and bulges at the equator due to its rotation. This makes the Earth an oblate spheroid (a type of ellipsoid). In an ellipsoid:

• The semi-major axis aaa is the radius at the equator (the longest radius).
• The semi-minor axis bbb is the radius from the center to the poles (the shortest radius).

Resources

• https://www.youtube.com/watch?v=kXTHaMY3cVk

1. Semi-major axis (a)

• This is the longest radius of the ellipse, which extends from the center of the ellipse to the farthest point on the surface. It is usually denoted by aaa and represents the equatorial radius of the ellipsoid.

2. Semi-minor axis (b)

• This is the shortest radius of the ellipse, extending from the center of the ellipse to the nearest point on the surface. It is usually denoted by bbb and represents the polar radius of the ellipsoid. The semi-minor axis can be derived from the semi-major axis and the flattening.

3. Flattening (f)

• Flattening quantifies how much the ellipse is “squashed” relative to a perfect sphere. It is calculated as: