Frequency Response

$H(jw)$ is called the frequency response, where $H(s)$ is simply the Transfer Function.

This feels so random, where did jw come from?

$s$ simply represents a complex number, which we can write $s=\sigma +j\omega$. So here, we are only interested in the imaginary part.

$H(j\omega )=|H(j\omega )|e^{j{\angle }_{H(j\omega )}}$

Theorem 1

If $S:f\to y$ is a LTI then for any $s\in \mathbb{C}$ $e^{st}\to H(s)e^{st}$

• Taken from LTI System page

Theorem 2

If $S:f\to y$ is a LTI then

$\sin (\omega t)\to |H(j\omega )|\sin (\omega t+{\angle }_{H(j\omega )})$

Theorem 3

If $S$ is a stable LTI with transfer function $H(s)$, then as $t\to \infty$

$\sin (\omega t)u(t)\to |H(j\omega )|\sin (\omega t+{\angle }_{H(j\omega )})$

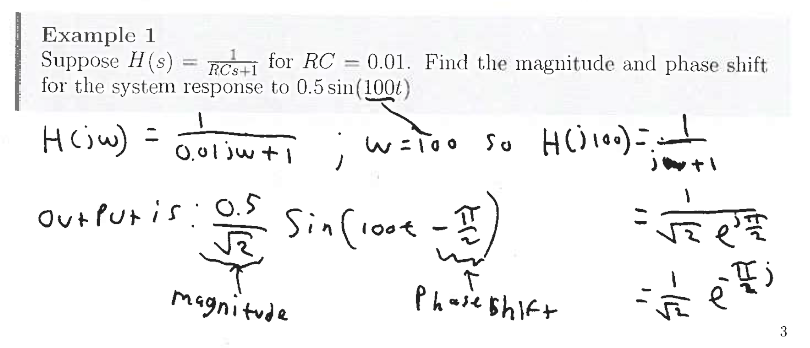
Other

• The frequency response of a system is the Fourier transform of its Impulse Response

If you feed a sinusoid into an LTI system, the output is also a sinusoid of the same frequency. The system may:

• Change the amplitude of the sinusoid.
• Shift the phase (delay/advance in time).

SE380

The frequency response of a system is the complex-valued function $G(j\omega )$. $G:\omega \in \mathbb{R}\to G(j\omega )\in \mathbb{C}$ which could be equivalently expressed as the following two real-valued functions (magnitude and phase responses): $|G|:\omega \in \mathbb{R}\to |G(j\omega )|\in \mathbb{R}$ $\angle G:\omega \in \mathbb{R}\to \angle G(j\omega )\in \mathbb{R}$

This function plays a fundamental role in the computation of the steady-state output response of a system to a sinusoidal input. Consider a system whose poles have negative real part. Given an input signal u(t) = U sin(ωt) and following the steps on Page 6 to compute the corresponding output response y(t), we get:

$y(t)=transient+|G(jw)|Usin(wt+<G(jw))$

Related

• Bode Plot