Integrals

Integral calculus is actually much older than differential calculus.

I find integrals harder than Derivatives. This is because there is no precise approach to solve all integrals, and so determining which technique to use at a given time takes experience. On the other hand, there are only a few rules for derivatives.

Definition of the Definite Integral

Let $f$ be continuous on the interval $[a,b]$. Partition $[a,b]$ into $n$ subintervals of equal length $\Delta x=\frac{b-a}{n}$. Label the endpoints of the subintervals $x_i$, for $i=0\dots n$ (so that the ith interval is $[x_{i-1},x_i]i=1\dots n$), and in each interval, select a point $x_i^{\ast }$. The definite integral of $f$ from $a$ to $b$ is

$Area={\int }_a^{b}f(x)dx={\lim }_{n\to \infty }{\sum }_{i=1}^{n}f(x_i^{\ast })\Delta x$

$x_i^{\ast }=a+i\Delta x$ where $\Delta x=\frac{b-a}{n}$

${\sum }_{i=1}^{n}f(x_i^{\ast })\Delta x$ is called a Riemann Sum

Integration Formulas

Definite Integrals

${\int }_a^{b}kdx=k(b-a)$ ${\int }_a^{a}f(x)dx=0$ ${\int }_a^b[f(x)\pm g(x)]dx={\int }_a^{b}f(x)dx\pm {\int }_a^{b}g(x)dx$ ${\int }_a^{b}kf(x)dx=k{\int }_a^{b}f(x)dx$ ${\int }_a^{b}f(x)dx=-{\int }_b^{a}f(x)dx$ ${\int }_a^{c}f(x)dx={\int }_a^{b}f(x)dx+{\int }_b^{c}f(x)dx$

It is permissible to integrate inequalities. If $f(x)\ge g(x)$ for $x\in [a,b]$, then ${\int }_a^{b}f(x)dx\ge {\int }_a^{b}g(x)dx$

Indefinite Integrals

$\int x^{n}dx=\frac{x^{n+1}}{n+1}+C,\text{for any number }n\ne -1$ $\int \frac{1}{x}dx=\ln |x|+C$ $\int e^{x}dx=e^x+C$ $\int a^{x}dx=\frac{a^x}{\ln (a)}+C$ $\int \cos xdx=\sin x+C$ $\int \sin xdx=-\cos x+C$ $\int {\sec }^{2}xdx=\tan x+C$ $\int {\csc }^{2}xdx=-\cot x+C$ $\int \sec x\tan xdx=\sec x+C$ $\int \csc x\cot xdx=-\csc x+C$ $\int \cosh xdx=\sinh x+C$ $\int \sinh xdx=\cosh x+C$ $\int \frac{1}{1+x^2}dx={\tan }^{-1}x+C$ $\int \frac{1}{\sqrt{1-x^2}}dx={\sin }^{-1}x+C$ $\int -\frac{1}{\sqrt{1-x^2}}dx={\cos }^{-1}x+C$ $\int \frac{1}{a^2+x^2}dx=\frac{1}{a}{\tan }^{-1}(\frac{x}{a})+C$

Below, we cover the techniques used.

Important Integrals

For the integrals, ${\int }_a^{b}f(x)dx$ and ${\int }_a^{b}f(x)dx$

If $b-a=m\pi$, where $m$ is an integer, then the value of the integrals is always $\frac{m\pi }{2}$

For the integral: ${\int }_a^b\sin (x)\cos (x)dx$

If $b-a=m\pi$, where $m$ is an integer, then the value of the integral is 0.

Integration Techniques

In a sense, there are only 4:

• The Method of Substitution (pretty straightforward)
  • includes Trigonometric Substitution
• Integration by Parts (IBP)
• Rewriting the integrand (by simple algebra, trig identities, partial fractions, or completing squares)

Applications of Integration

Areas Between Curves

$\text{Area}={\int }_a^b[f(x)-g(x)]dx$

Sometimes, we use $y$ as the variable of integration to make it easier to calculate.

Finding Lengths of Curves

$L={\int }_a^b\sqrt{1+(\frac{dy}{dx}{)}^2}dx$

Mean Values of Functions

$m.v.(f)=\frac{1}{b-a}{\int }_a^{b}f(x)dx$

For a multivariate function $f(x,y)$, $f_{avg}=\frac{1}{Area}{\iint }_{R}f(x,y)dA$

Volumes of Solids of Revolution

I always struggled with this topic.

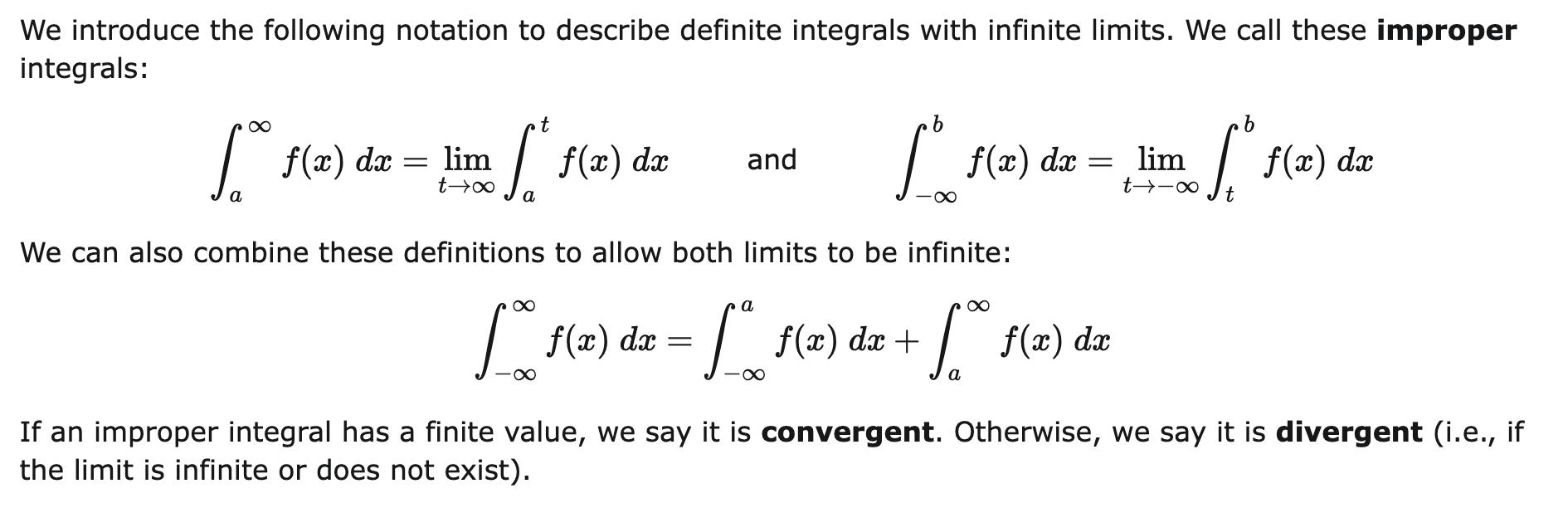
Improper Integral