Convex Optimization

Professor Yash Pant talked about this.

Noam Brown also talks about this.

• Course by Stanford: https://see.stanford.edu/Course/EE364A

Also heard about this from the Ericsson guy I played golf with, when he was doing his PhD.

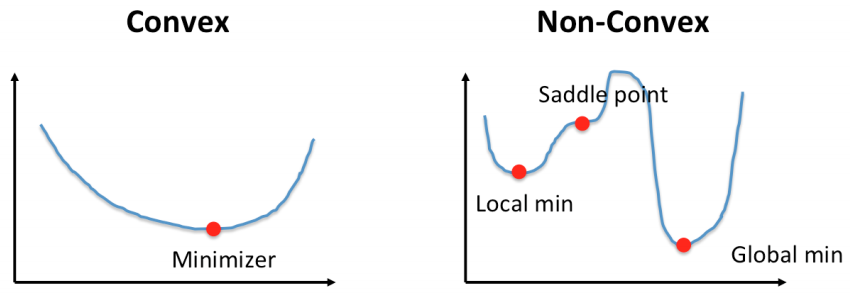
Soham also explained this to me. But basically, it’s the idea that there exists a longest point for a given function. Having convex functions give these really nice properties which allows you to solve them more easily.

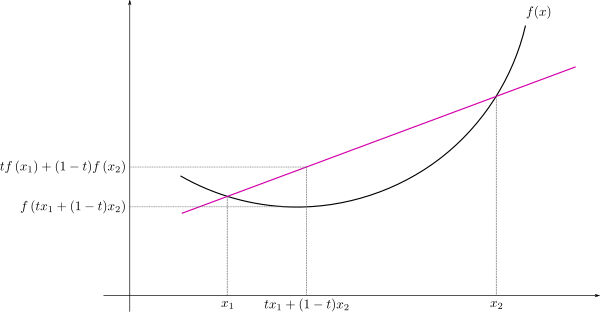
Convex Function: a real-valued function is called convex if the line segment between any two points on the graph of the function lies above the graph between the two points.

If you draw a line between any two points, the curve will be below the line.