Laplace Transform

Given a function $f(t)$, the Laplace Transform of $f(t)$ denoted by $F(s)$ (given that the integral exists) is defined by

$F(s)=\mathcal{L}\{f(t)\}={\int }_{-\infty }^{\infty }f(t)e^{-st}dt$

• $s$ is a complex parameter called the frequency

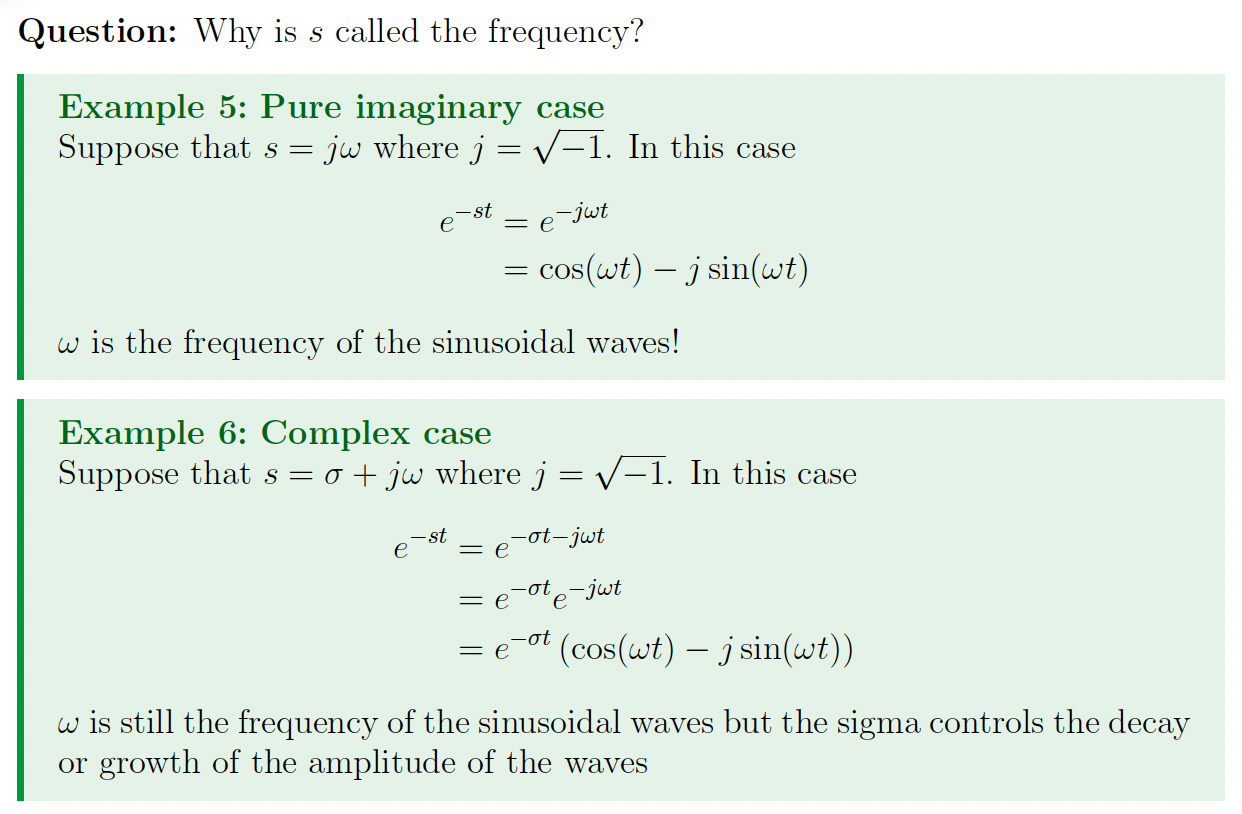
Why is s called the frequency?

In $s=\sigma +j\omega$, the term $\omega$ relates to angular frequency in radians per second.

The teacher gives these two examples:

Laplace Transforms of common functions:

• https://lpsa.swarthmore.edu/LaplaceXform/FwdLaplace/LaplaceFuncs.html

Properties of Laplace Transform

Theorem 1

For any function $f(t)$, the one-sided Laplace transform will always converge for some $s$ that $Re(s)$ is sufficiently large.

Theorem 2: Laplace Transform is Linear

Suppose that $f(t)$ and $g(t)$ have Laplace transforms $F(s)$ and $G(s)$. Then for all $\alpha ,\beta \in C$

$\mathcal{L}\{\alpha f(t)+\beta g(t)\}=\alpha F(s)+\beta G(s)$ the the ROC is the intersection of the ROCs for $F(s)$ and $G(s)$.

Theorem 3: Time-Scaling

If $\mathcal{L}\{f(t)\}=F(s)$ then for $c>0,\mathcal{L}\{f(ct)\}=\frac{1}{c}F(\frac{s}{c})$

Theorem 4: Exponential Modulation

$\mathcal{L}\{e^{\alpha t}f(t)\}=F(s-\alpha )$

Theorem 5: Time-Shifting

If $F(s)=\mathcal{L}\{f(t)u(t)\}$ and $g(t)=f(t-T)u(t-T)$ then

$G(s)=e^{-sT}F(s)$

Theorem 6: Multiplication by t

If $\mathcal{L}\{f(t)\}=F(s)$ then $\mathcal{L}\{tf(t)\}=-\frac{d}{ds}F(s)$

Theorem 7: Laplace Transform of a Derivative/Integral

Let $f(t)$ be such that there is a real value α such that the integral ${\int }_{0^{-}}^{\infty }|f(t)|e^{-\alpha t}dt$

converge and such that there exists a function $f^{\prime }(t)$ such that for $t\ge 0$ $f(t)=f(0^{-})+{\int }_{0^{-}}^{\infty }{f}^{\prime }(\tau )d\tau$

and there exists a real value $\beta$ such that ${\int }_{0^{-}}^{\infty }|f^{\prime }(t)|e^{-\beta t}dt$

converges. In this case $F(s)=\frac{1}{s}f(0^{-})+\frac{1}{s}\mathcal{L}\{f^{\prime }(t)\}$ or in other-words $\mathcal{L}\{f^{\prime }(t)\}=sF(s)-f(0^{-})$

• We can use this theorem to solve linear ODEs!

More Laplace Transforms

• Inverse Laplace Transform
• Unilateral Laplace Transform