Power Series

A power series centered at $x_0$ is a series of the form ${\sum }_{n=0}^{\infty }{c}_n(x-x_0{)}^n=c_0+c_1(x-x_0)+c_2(x-x_0{)}^2+\cdots$

A Taylor Polynomials is just a form of a Power Series!

Radius of Convergence

Note

Every power series $F(x)={\sum }_{n=0}^{\infty }{a}_n(x-c{)}^n$ has a radius of convergence $R$, which is either a nonnegative number ($R\ge 0$) or infinity ($R=\infty$). If $R$ is finite,

• $F(x)$ converges absolutely when $|x-c|<R$
• $F(x)$ diverges when $|x-c|>R$.

If $R=\infty$, then $F(x)$ converges absolutely for all $x$.

$\text{Radius of Convergence}=\frac{1}{2}\cdot \text{Interval of Convergence}$

Steps for finding the Interval of Convergence

1. Apply the ratio test to find the radius of converge $r$.
2. Check the endpoints (to determine whether we use ( or [) → because the ratio test fails at $L=1$

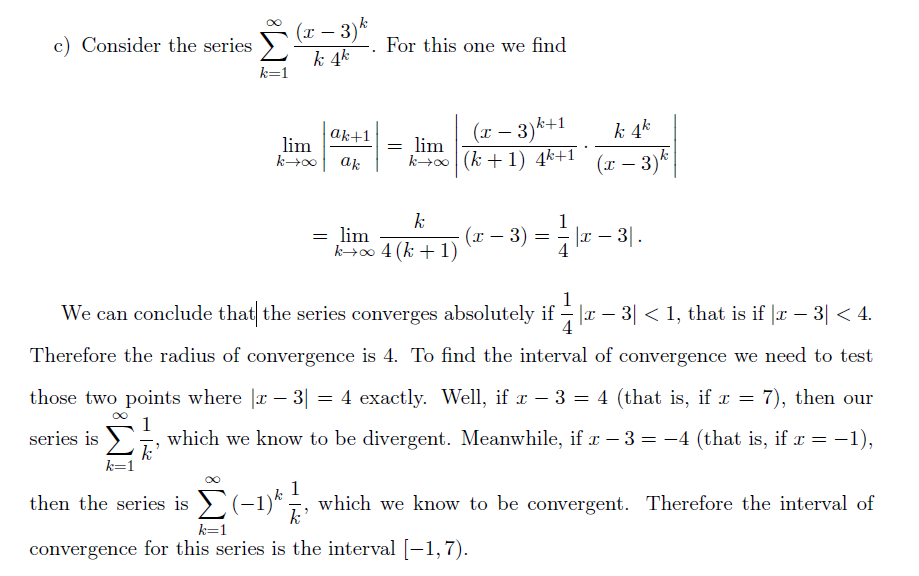
Example:

You can approximate PI!

arctanx

Tricks

Manipulations for Radius of Convergence R

Theorem: If the series $\sum (x-x_0{)}^k$ has radius of convergence $R$, then we can:

• differentiate it (term-by-term)
• integrate it (term-by-term)
• multiply through by a constant (term-by-term)
• add it (term-by-term) to another series of radius of convergence ≥ R

and the result will also have radius of convergence $R$.

Basic Building Blocks for Maclaurin Series

$\frac{1}{1-x}={\sum }_{k=0}^{\infty }{x}^k,\text{converges for }|x|<1$ $e^x={\sum }_{k=0}^{\infty }\frac{x^k}{k!},\text{converges for all }x$

$\sin x={\sum }_{x=0}^{\infty }(-1{)}^k\frac{x^{2k+1}}{(2k+1)!},\text{converges for all }x$ $\cos x={\sum }_{x=0}^{\infty }(-1{)}^k\frac{x^{2k}}{(2k)!},\text{converges for all }x$

$\ln (1+x)={\sum }_{k=0}^{\infty }\frac{(-1{)}^{k-1}{x}^k}{k},\text{converges for }|x|<1\text{ and }x=1$ ${\tan }^{-1}x={\sum }_{k=0}^{\infty }\frac{(-1{)}^k{x}^{2k+1}}{2k+1},\text{converges for }|x|\le 1$ $(1+x{)}^a={\sum }_{k=0}^{\infty }(\genfrac{}{}{0ex}{}{a}{k})x^k,\text{converges for }|x|<1$

Next

• You can use these power series in Big-O Notation